Considering Meanders and Labyrinths

In a series of recent posts Erwin has elaborated on meanders in labyrinths. He has found a meander best suited for labyrinths. This particular meander exists in various forms. Erwin refers to these as “type” followed by an even number, e.g. type 4, type 6 and so forth.

Indeed, these types of meanders can be often found in existing labyrinths. However other figures occur in labyrinths too that can be denoted as meanders. Generally a broad range of figures is referred to as meanders. And it seems not so clear what a meander is at all.

So, what is a meander?

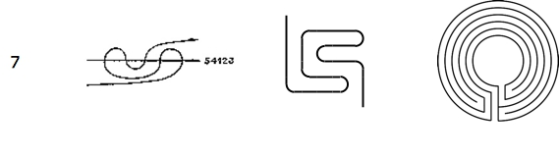
On his website, Tony Phillips cites Arnol’d, a Russian mathematician. Independent of the labyrinth, Arnol’d wanted to investigate how many meanders there are. He defines a meander as follows:

• Connected oriented curve,
• that does not intersect itself
• and intersects a fixed, oriented line in several points.

He illustrates this with the example of a curve that intersects the fixed line five times. He found that there exist eight different such curves. I have copied the illustration from Tony’s website, enumerated the 8 curves and display it below.

8 curves

It can easily be recognized that these curves are very closely related with the labyrinth. One only has to rotate them by a quarter of a circle in clockwise direction and straighten them out somewhat. This results in the patterns of 8 one-arm labyrinths with 5 circuits each. Therefore, let us have a closer look at these figures.

figure 1

This figure represents a serpentine that leads from the entrance to the center of the labyrinth. It is this the pattern of the historical Näpfchenstein labyrinth. This labyrinth is self-dual.

figure 2

This curve depicts the pattern of the labyrinth type Löwenstein 5b. This is the dual of the labyrinth shown in figure 4. Dual labyrinths have the same pattern, although the pattern is rotated by half a circle, and the entrance and center are exchanged. The “Rockery Labyrinth“, designed by Erwin is also of this type.

figure 3

This curve includes a pattern of the Knossos type (3 circuits) to which are attached an additional circuit on both, the outer and the inner side. I am not aware of any existing labyrinth of this type. This labyrinth is self-dual.

figure 4

This is the pattern of the labyrinth type Löwenstein 5a. It is dual to the labyrinth shown in figure 2. The “Pilgrim Hospices” labyrinth, designed by The Labyrinth Builders is of this type.

figure 5

This figure contains the pattern of the labyrinth I use for my investigations and presentations, so to speak my demonstration labyrinth. It has the following properties that are important for this purpose: the path does not enter on the first circuit, it does not reach the center from the last circuit and the labyrinth is not self-dual. The dual to this labyrinth is shown in figure 7.

figure 6

This pattern corresponds with a serpentine from the inside out. The path enters along the axis and first encounters the innermost circuit. From there it winds itself out circuit by circuit until it reaches the first (outermost) circuit. Then it is directed axially to the center. Erwin has discovered this type of labyrinth (Chartres 5 classical) by omitting the side-arms of the Compiègne-type labyrinth. This labyrinth is self-dual.

figure 7

This is the dual of my demonstration labyrinth shown in figure 5.

figure 8

This curve represents Erwin’s meander best suited for labyrinths. It is a type 6 meander. This is the pattern of the core-labyrinth of the historical Rockcliffe Marsh labyrinth. Rockcliffe Marsh is a very special labyrinth. First it has an unusual layout. The figure is opened along the axis and unrolled to a segment of a circle. Second, it is made up of a core-labyrinth (the inner 5 circuits) that is enclosed by a spiral outside.

Conclusion

Arnol’d’s definition of a meander is closely related with the labyrinth. His curves correspond with the patterns of all one-arm labyrinths in which the pathway does not cross the axis. The number of intersections between the curve and the fixed line corresponds with the number of circuits in the labyrinth. This was demonstrated in detail for labyrinths with 5 circuits.

• Thus there exist 8 different patterns for a labyrinth with one axis and five circuits with the pathway not crossing the axis.
• With an increasing number of circuits, the number of different pattern increases dramatically. E.G. there are 14 possible patterns for a labyrinth with 6 and 42 for a labyrinth with 7 circuits.
• According to Arnol’d’s definition, all 8 figures are meanders. If we follow Erwin’s definition, only figure 8 is a meander suited for labyrinths.
• If we adopt the definition of Erwin, we will capture the most common and essential labyrinths. However, we will also miss a broad range of existing and potential patterns of labyrinths.
• If we adopt the definition of Arnol’d, every pattern of a one-arm labyrinth, in which the way does not cross the axis is referred to as a meander. This definition seems too broad and can be further differentiated.